DC motors
A simple DC motor has a coil of wire that can rotate in a magnetic field. The
current in the coil is supplied via two brushes that make moving contact with
a split ring. The coil lies in a steady magnetic field. The forces exerted
on the current-carrying wires create a torque on the coil.
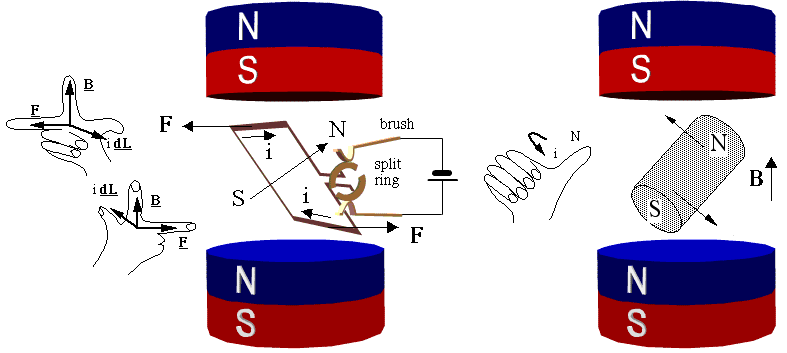
The force F on a wire of length L carrying a current i in a magnetic field
B is iLB times the sine of the angle between B and i, which would be 90° if
the field were uniformly vertical. The direction of F comes from the right
hand rule*, as shown here. The two forces shown here are equal and opposite,
but they are displaced vertically, so they exert a torque. (The forces on
the other two sides of the coil act along the same line and so exert no torque.)
-
*
A number of different nmemonics are used to remember the direction of
the force. Some use the right hand, some the left. For students who
know vector multiplication, it is easy to use the Lorentz force directly: F = q v X B , whence F = i dL X B . That is the origin of the diagram shown here.
The coil can also be considered as a magnetic dipole, or a little electromagnet,
as indicated by the arrow SN: curl the fingers of your right hand in the
direction of the current, and your thumb is the North pole. In the sketch
at right, the electromagnet formed by the coil of the rotor is represented
as a permanent magnet, and the same torque (North attracts South) is seen
to be that acting to align the central magnet.
-
Throughout, we use blue for the North pole and
red for the South. This is just a convention to make the orientation
clear: there is no difference in the material at either end of the
magnet, and they are usually not painted a different colour.
Note the effect of the brushes on the split ring. When the
plane of the rotating coil reaches horizontal, the brushes will break contact
(not much is lost, because this is the point of zero torque anyway – the forces
act inwards). The angular momentum of the coil carries it past this break
point and the current then flows in the opposite direction, which reverses
the magnetic dipole. So, after passing the break point, the rotor continues
to turn anticlockwise and starts to align in the opposite direction. In the
following text, I shall largely use the 'torque on a magnet' picture, but
be aware that the use of brushes or of AC current can cause the poles of
the electromagnet in question to swap position when the current changes direction.
The torque generated over a cycle varies with the vertical separation of
the two forces. It therefore depends on the sine of the angle between the
axis of the coil and field. However, because of the split ring, it is always
in the same sense. The animation below shows its variation in time, and you
can stop it at any stage and check the direction by applying the right hand
rule.
Motors and generators
Now a DC motor is also a DC generator. Have a look at the next animation. The
coil, split ring, brushes and magnet are exactly the same hardware as the motor
above, but the coil is being turned, which generates an emf.
If you use mechanical energy to rotate the coil (N turns, area A) at uniform
angular velocity ω in the magnetic field B,
it will produce a sinusoidal emf in the coil. emf (an emf or
electromotive force is almost the same thing as a voltage). Let θ be
the angle between B and the normal to the coil, so the magnetic flux φ is
NAB.cos θ. Faraday's law gives:
-
emf = − dφ/dt = − (d/dt)
(NBA cos θ)
= NBA sin θ (dθ/dt)
= NBAω sin ωt.
The animation above would be called a DC generator. As in the DC motor,
the ends of the coil connect to a split ring, whose two halves are contacted
by the brushes. Note that the brushes and split ring 'rectify' the emf produced:
the contacts are organised so that the current will always flow in the same
direction, because when the coil turns past the dead spot, where the brushes
meet the gap in the ring, the connections between the ends of the coil and
external terminals are reversed. The emf here (neglecting the dead spot, which conveniently happens at zero volts) is
|NBAω sin ωt|,
as sketched.
An alternator
If we want AC, we don't need recification, so we don't need split rings. (This
is good news, because the split rings cause sparks, ozone, radio interference and extra wear. If you want
DC, it is often better to use an alternator and rectify with diodes.)
In the next animation, the two brushes contact two continuous rings, so
the two external terminals are always connected to the same ends of the coil.
The result is the unrectified, sinusoidal emf given by NBAω sin ωt,
which is shown in the next animation.
This is an AC generator. The advantages of AC and DC
generators are compared in a section below. We saw above that a DC motor
is also a DC generator. Similarly, an alternator is also an AC motor. However,
it is a rather inflexible one. )
Back emf
Now, as the first two animations show, DC motors and generators may be the
same thing. For example, the motors of trains become generators when the train
is slowing down: they convert kinetic energy into electrical energy and put
power back into the grid. Recently, a few manufacturers have begun making motor cars
rationally. In such cars, the electric motors used to drive the car are also
used to charge the batteries when the car is stopped - it is called regenerative
braking.
So here is an interesting corollary. Every motor is a generator. This is
true, in a sense, even when it functions as a motor. The emf that a motor
generates is called the back emf.
The back emf increases with the speed, because of Faraday's law. So, if
the motor has no load, it turns very quickly and speeds up until the
back emf, plus the voltage drop due to losses, equal the supply
voltage. The back emf can be thought of as a 'regulator': it stops the
motor turning infinitely quickly (thereby saving physicists some
embarrassment). When the motor is loaded, then the phase of the voltage
becomes closer to that of the current (it starts to look resistive) and
this apparent resistance gives a voltage. So the back emf required is
smaller, and the motor turns more slowly. (To add the back emf, which
is inductive, to the resistive component, you need to add voltages that
are out of phase.
Coils usually have cores
In practice, (and unlike the diagrams we have drawn), generators and DC
motors often have a high permeability core inside the coil, so that large
magnetic fields are produced by modest currents. This is shown at left in
the figure below in which the stators (the magnets which are stat-ionary)
are permanent magnets.
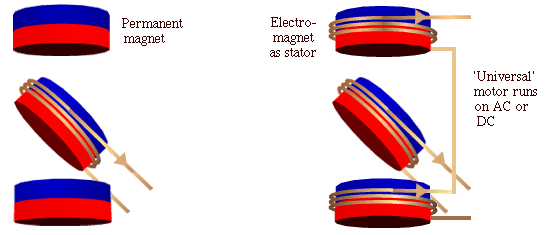
'Universal' motors
The stator magnets, too, could be made as electromagnets, as is shown above
at right. The two stators are wound in the same direction so as to give a
field in the same direction and the rotor has a field which reverses twice
per cycle because it is connected to brushes, which are omitted here. One
advantage of having wound stators in a motor is that one can make a motor
that runs on AC or DC, a so called universal motor.
When you drive such a motor with AC, the current in the coil changes
twice in each cycle (in addition to changes from the brushes), but the
polarity of the stators changes at the same time, so these changes
cancel out.
Build a simple motor
To build this simple but strange motor, you need two fairly strong magnets
(rare earth magnets about 10 mm diameter would be fine, as would larger bar
magnets), some stiff copper wire (at least 50 cm), two wires with crocodile
clips on either end, a six volt lantern battery, two soft drink cans, two blocks
of wood, some sticky tape and a sharp nail.
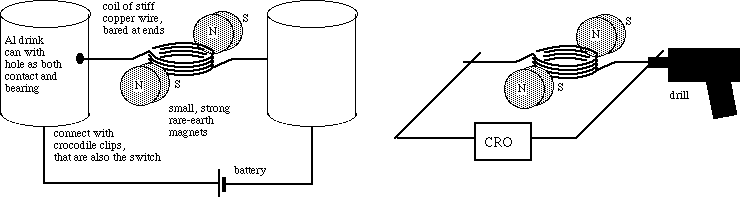
Make the coil out of stiff copper wire, so it doesn't need any external
support. Wind 5 to 20 turns in a circle about 20 mm in diameter, and have
the two ends point radially outwards in opposite directions. These ends will
be both the axle and the contacts. If the wire has lacquer or plastic insulation,
strip it off at the ends.
An even simpler motor (one that is also much simpler to understand!) is the homopolar motor.
AC motors
With AC currents, we can reverse field directions without having to use brushes.
This is good news, because we can avoid the arcing, the ozone production and
the ohmic loss of energy that brushes can entail. Further, because brushes
make contact between moving surfaces, they wear out.
The first thing to do in an AC motor is to create a rotating field. 'Ordinary'
AC from a 2 or 3 pin socket is single phase AC--it has a single sinusoidal
potential difference generated between only two wires--the active and neutral.
(Note that the Earth wire doesn't carry a current except in the event of
electrical faults.) With single phase AC, one can produce a rotating field
by generating two currents that are out of phase using for example a capacitor.
In the example shown, the two currents are 90° out of phase, so the vertical
component of the magnetic field is sinusoidal, while the horizontal is cosusoidal,
as shown. This gives a field rotating counterclockwise.
(* I've been asked to explain this: from simple AC
theory, neither coils nor capacitors have the voltage in phase with
the current. In a capacitor, the voltage is a maximum when the charge has
finished flowing onto the capacitor, and is about to start flowing off.
Thus the voltage is behind the current. In a purely inductive coil, the
voltage drop is greatest when the current is changing most rapidly, which
is also when the current is zero. The voltage (drop) is ahead of the current.
In motor coils, the phase angle is rather less than 90¡, because electrical
energy is being converted to mechanical energy.)
In this animation, the graphs show the variation in time of the currents
in the vertical and horizontal coils. The plot of the field components Bx and
By shows that the vector sum of these two fields is a rotating
field. The main picture shows the rotating field. It also shows the polarity
of the magnets: as above, blue represents a North pole and red a South pole.
If we put a permanent magnet in this area of rotating field, or if we put
in a coil whose current always runs in the same direction, then this becomes
a synchronous motor. Under a wide range of conditions, the motor will
turn at the speed of the magnetic field. If we have a lot of stators, instead
of just the two pairs shown here, then we could consider it as a stepper
motor: each pulse moves the rotor on to the next pair of actuated poles.
Please remember my warning about the idealised geometry: real stepper motors
have dozens of poles and quite complicated geometries!
Induction motors
Now, since we have a time varying magnetic field, we can use the induced emf
in a coil – or even just the eddy currents in a conductor – to make the rotor
a magnet. That's right, once you have a rotating magnetic field, you can just
put in a conductor and it turns. This gives several of the advantages of
induction motors: no brushes or commutator means easier manufacture, no
wear, no sparks, no ozone production and none of the energy loss associated
with them. Below left is a schematic of an induction motor.
The animation at right represents a squirrel cage motor. The squirrel
cage has (in this simplified geometry, anyhow!) two circular conductors joined
by several straight bars. Any two bars and the arcs that join them form a
coil – as indicated by the blue dashes in the animation. (Only two of the
many possible circuits have been shown, for simplicity.)
This schematic suggests why they might be called squirrel cage motors.
The reality is different: for photos and more details. The problem with the induction and squirrel cage motors shown
in this animation is that capacitors of high value and high voltage rating
are expensive. One solution is the 'shaded pole' motor, but its rotating
field has some directions where the torque is small, and it has a tendency
to run backwards under some conditions. The neatest way to avoid this is
to use multiple phase motors.
Three phase AC induction motors
Single phase is used in domestic applications for low power applications but
it has some drawbacks. One is that it turns off 100 times per second (you don't
notice that the fluorescent lights flicker at this speed because your eyes
are too slow: even 25 pictures per second on the TV is fast enough to give
the illusion of continuous motion.) The second is that it makes it awkward
to produce rotating magnetic fields. For this reason, some high power (several
kW) domestic devices may require three phase installation. Industrial applications
use three phase extensively, and the three phase induction motor is a standard
workhorse for high power applications. The three wires (not counting earth)
carry three possible potential differences which are out of phase with each
other by 120°, as shown in the animation below. Thus three stators give a smoothly
rotating field.
If one puts a permanent magnet in such a set of stators, it becomes a synchronous
three phase motor. The animation shows a squirrel cage, in which for
simplicity only one of the many induced current loops is shown. With no
mechanical load, it is turning virtually in phase with the rotating field.
The rotor need not be a squirrel cage: in fact any conductor that will
carry eddy currents will rotate, tending to follow the rotating field.
This arrangement can give an induction motor capable of high efficiency,
high power and high torques over a range of rotation rates.
Linear motors
A set of coils can be used to create a magnetic field that translates, rather
than rotates. The pair of coils in the animation below are pulsed on, from
left to right, so the region of magnetic field moves from left to right. A
permanent or electromagnet will tend to follow the field. So would a simple
slab of conducting material, because the eddy currents induced in it (not shown)
comprise an electromagnet. Alternatively, we could say that, from Faraday's
law, an emf in the metal slab is always induced so as to oppose any change
in magnetic flux, and the forces on the currents driven by this emf keep the
flux in the slab nearly constant. (Eddy currents not shown in this animation.)
Alternatively, we could have sets of powered coils in the moving part,
and induce eddy currents in the rail. Either case gives us a linear motor,
which would be useful for say maglev trains. (In the animation, the geometry
is, as usual on this site, highly idealised, and only one eddy current is
shown.)
Some notes about AC and DC motors for high power applications
-
This site was originally written to help high school students
and teachers in New South Wales, Australia, where a new syllabus concentrating
on the history and applications of physics, at the expense of physics itself,
has been introduced. The new syllabus, in one of the dot points, has this
puzzling requirement: "explain that AC motors usually produce low power and
relate this to their use in power tools".
AC motors are used for high power applications whenever it is possible. Three
phase AC induction motors are widely used for high power applications, including
heavy industry. However, such motors are unsuitable if multiphase is unavailable,
or difficult to deliver. Electric trains are an example: it is easier to build
power lines and pantographs if one only needs one active conductor, so this
usually carries DC, and many train motors are DC. However, because of the disadvantages
of DC for high power, more modern trains convert the DC into AC and then run
three phase motors.
Single phase induction motors have problems for applications combining
high power and flexible load conditions. The problem lies in producing the
rotating field. A capacitor could be used to put the current in one set of
coils ahead, but high value, high voltage capacitors are expensive. Shaded
poles are used instead, but the torque is small at some angles. If one cannot
produce a smoothly rotating field, and if the load 'slips' well behind the
field, then the torque falls or even reverses.
Power tools and some appliances use brushed AC motors. Brushes introduce
losses (plus arcing and ozone production). The stator polarities are reversed
100 times a second. Even if the core material is chosen to minimise hysteresis
losses ('iron losses'), this contributes to inefficiency, and to the possibility
of overheating. These motors may be called 'universal'
motors because they can operate on DC. This solution is cheap, but crude
and inefficient. For relatively low power applications like power tools,
the inefficiency is usually not economically important.
If only single phase AC is available, one may rectify the AC and use a
DC motor. High current rectifiers used to be expensive, but are becoming
less expensive and more widely used. If you are confident you understand
the principles, it's time to go to How
real electric motors work by John Storey. Or else continue here to find
out about loudspeakers and transformers.
Loudspeakers
A loudspeaker is a linear motor with a small range. It has a single moving
coil that is permanently but flexibly wired to the voltage source, so there
are no brushes.
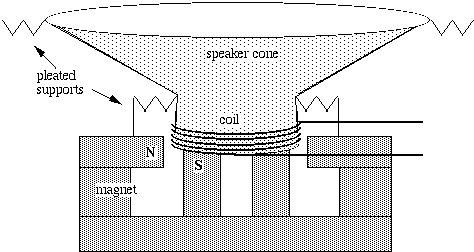 The
coil moves in the field of a permanent magnet, which is usually shaped
to produce maximum force on the coil. The moving coil has no core, so
its mass is small and it may be accelerated quickly, allowing for high
frequency motion. In a loudspeaker, the coil is attached to a light weight
paper cone, which is supported at the inner and outer edges by circular,
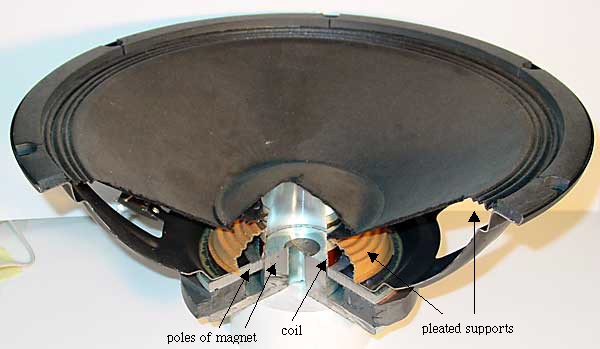
pleated paper 'springs'. In the photograph below, the speaker is beyond
the normal upward limit of its travel, so the coil is visible above the
magnet poles.
For low frequency, large wavelength sound, one needs large cones.
The speaker shown below is 380 mm diameter. Speakers designed for
low frequencies are called woofers. They have large mass and are
therefore difficult to accelerate rapidly for high frequency sounds.
In the photograph below, a section has been cut away to show the
internal components. The
coil moves in the field of a permanent magnet, which is usually shaped
to produce maximum force on the coil. The moving coil has no core, so
its mass is small and it may be accelerated quickly, allowing for high
frequency motion. In a loudspeaker, the coil is attached to a light weight
paper cone, which is supported at the inner and outer edges by circular,
pleated paper 'springs'. In the photograph below, the speaker is beyond
the normal upward limit of its travel, so the coil is visible above the
magnet poles.
For low frequency, large wavelength sound, one needs large cones.
The speaker shown below is 380 mm diameter. Speakers designed for
low frequencies are called woofers. They have large mass and are
therefore difficult to accelerate rapidly for high frequency sounds.
In the photograph below, a section has been cut away to show the
internal components.
Tweeters - loudspeakers designed for high frequencies - may be just speakers of similar design, but with small, low mass cones and coils. Alternatively, they may use piezoelectric crystals to move the cone. |
Speakers are seen to be linear motors with a modest range - perhaps tens of
mm. Similar linear motors, although of course without the paper cone, are often
used to move the reading and writing head radially on a disc drive.
Loudspeakers as microphones
In the picture above, you can see that a cardboard diaphragm (the
loudspeaker cone) is connected to a coil of wire in a magnetic field.
If a soundwave moves the diaphragm, the coil will move in the field,
generating a voltage. This is the principle of a dynamic microphone –
though in most microphones, the diaphragm is rather smaller than the
cone of a loudspeaker. So, a loudspeaker should work as a microphone.
This is a nice project: all you need is a loudspeaker and two wires to
connect it to the input of an oscilloscope or the microphone input of
your computer. Two questions: what do you think the mass of the cone
and coil will do to the frequency response? What about the wavelength
of sounds your use?
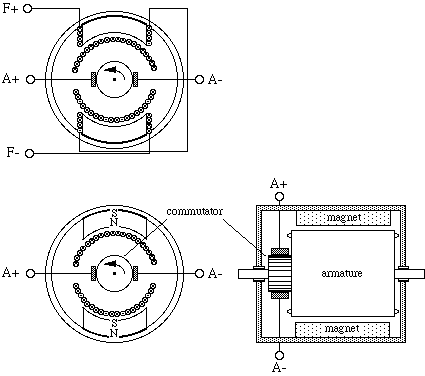
Warning: real motors are more complicatedThe sketches of motors have been schematics to show the principles. Please don't be angry if, when you pull a motor apart, it looks more complicated! For instance, a typical DC motor is likely to have many separately wound coils to produce smoother torque: there is always one coil for which the sine term is close to unity. This is illustrated below for a motor with wound stators (above) and permanent stators (below). |
Transformers
The photograph shows a transformer designed for demonstration purposes:
the primary and secondary coils are clearly separated, and may be removed
and replaced by lifting the top section of the core. For our purposes, note
that the coil on the left has fewer coils than that at right (the insets
show close-ups).

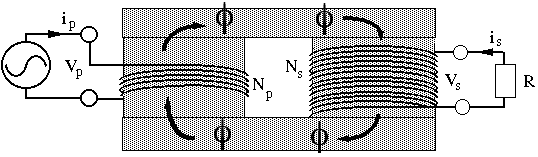
The sketch and circuit show a step-up transformer. To make a step-down transformer,
one only has to put the source on the right and the load on the left. (Important
safety note: for a real transformer, you could only 'plug it in backwards'
only after verifying that the voltage rating were appropriate.) So, how does
a transformer work?
The core (shaded) has high magnetic permeability, ie a material that forms
a magnetic field much more easily than free space does, due to the orientation
of atomic dipoles. (In the photograph, the core is laminated soft iron.)
The result is that the field is concentrated inside the core, and almost
no field lines leave the core. If follows that the magnetic fluxes φ through
the primary and secondary are approximately equal, as shown. From Faraday's
law, the emf in each turn, whether in the primary or secondary coil, is −dφ/dt.
If we neglect resistance and other losses in the transformer, the terminal
voltage equals the emf. For the Np turns of the primary, this
gives
-
Vp = − Np.dφ/dt
.
For the Ns turns of the secondary, this gives
-
Vs = − Ns.φ/dt
Dividing these equations gives the transformer equation
-
Vs/Vp = Ns/Np = r.
where r is the turns ratio. What about the current? If we neglect losses in
the transformer (see the section below on efficiency), and if we assume that
the voltage and current have similar phase relationships in the primary and
secondary, then from conservation of energy we may write, in steady state:
Power in = power out, so
VpIp = VsIs, whence
Is/Ip = Np/Ns = 1/r.
So you don't get something for nothing: if you increase the voltage, you decrease
the current by (at least) the same factor. Note that, in the photograph, the
coil with more turns has thinner wire, because it is designed to carry less
current than that with fewer turns.
In some cases, decreasing the current is the aim of the exercise. In power
transmission lines, for example, the power lost in heating the wires due
to their non-zero resistance is proportional to the square of the current.
So it saves a lot of energy to transmit the electrical power from power station
to city at very high voltages so that the currents are only modest.
Finally, and again assuming that the transformer is ideal, let's ask what
the resistor in the secondary circuit 'looks like' to the primary circuit.
In the primary circuit:
-
Vp = Vs/r and Ip =
Is.r so
Vp/Ip = Vs/r2Is =
R/r2.
R/r2 is called the reflected resistance. Provided that the
frequency is not too high, and provided that there is a load resistance (conditions
usually met in practical transformers), the inductive reactance of the primary
is much smaller than this reflected resistance, so the primary circuit behaves
as though the source were driving a resistor of value R/r2.
Efficiency of transformers
In practice, real transformers are less than 100% efficient.
- First, there are resistive losses in the coils (losing power I2.r). For a given material, the resistance of the coils can be reduced by making their cross section large. The resistivity can also be made low by using high purity copper.
- Second, there are some eddy current losses in the core. These can be reduced by laminating the core. Laminations reduce the area of circuits in the core, and so reduce the Faraday emf, and so the current flowing in the core, and so the energy thus lost.
- Third, there are hysteresis losses in the core. The magentisation and demagnetisation curves for magnetic materials are often a little different (hysteresis or history depedence) and this means that the energy required to magnetise the core (while the current is increasing) is not entirely recovered during demagnetisation. The difference in energy is lost as heat in the core.
- Finally, the geometric design as well as the material of the core may be optimised to ensure that the magnetic flux in each coil of the secondary is nearly the same as that in each coil of the primary.
More about transformers: AC vs DC generators
Transformers only work on AC, which is one of the great advantages of AC. Transformers
allow 240V to be stepped down to convenient levels for digital electronics
(only a few volts) or for other low power applications (typically 12V). Transformers
step the voltage up for transmission, as mentioned above, and down for safe
distribution. Without transformers, the waste of electric power in distribution
networks, already high, would be enormous. It is possible to convert voltages
in DC, but more complicated than with AC. Further, such conversions are often
inefficient and/or expensive. AC has the further advantage that it can be used
on AC motors, which are usually preferable to DC motors for high power applications.
By using Car Rental 8 you can find the best rental cars at over 50000 locations worldwide.
ReplyDelete